The type III radio bursts are the most intense radio emissions from the sun. In Figure 1, we present a typical type III burst observed by the STEREO spacecraft. Ginzburg and Zheleznyakov (1958) were the first to suggest that Langmuir waves excited by the solar flare accelerated electrons are the source of these bursts. The in situ detection of electron beams and Langmuir waves in association with type III bursts together with tracking of type III burst sources in the interplanetary medium confirmed this plasma hypothesis. However, the dynamics of type III electron beams and their interaction with the ambient plasma through the excited Langmuir waves is an unsolved problem. In order that the electron beams don’t lose their energy by resonantly interacting with Langmuir waves, various nonlinear processes, such as the induced scattering of Langmuir waves off the background ions in weak turbulence regime and oscillating two-stream instability in strong turbulence regime were invoked. However, the observed electron density fluctuations do not allow Langmuir waves to grow to very high intensities as pointed out by several authors. As far as the conversion of Langmuir wave energy into electromagnetic energy is concerned, there is no consensus either for the fundamental or for the second harmonic emission. In this paper, we address these issues in terms of critical fluctuations near the boundary of the beam-plasma instability which is analogous to the anomalously growing fluctuations near the phase transition points called critical points. We show that this phenomenon can account for the survival of electron beams over large distances as well as the intensities of Langmuir waves excited during this phase transition regime are sufficient to explain the peak intensities of the fundamental as well as harmonic emissions.
Critical Fluctuations in the Beam-Plasma Systems
The equation for the effective temperature $T_{eff}$ of Langmuir waves excited in a layer of thickness $dl$ by the beam-plasma instability can be written as:
\[\frac {dT_{eff}}{dl} = \alpha – \mu T_{eff}, \]
where $\alpha$ and $\mu$ are the emission and absorption coefficients, respectively. The $\mu$ is determined by the absorption due to collisions $(\mu_c)$, effective absorption due to scattering by density fluctuations $(\mu_{sca}$ and negative absorption due to electron beam $(\mu_b)$:
\[ \mu = \mu_c + \mu_{sca} + \mu_b. \]
If the negative absorption due to electron beam is approximately balanced by the damping due either to collisions in the corona or to scattering on electron density fluctuations in the interplanetary medium, then $\mu \approx 0$ and: $T_{eff} \approx \alpha L$. For typical parameters, these conditions yield the ratio of beam to ambient electron density, $\frac {N_b}{N_e} = 5.23 \times 10^{-8}$ and $T_{eff} \simeq 10^{12}$ K for 100 MHz In the case of interplanetary type III bursts at 13 kHz, for $\frac{N_b}{N_e} \simeq 3.5 \times 10^{-5}$, we obtain $T_{eff} \simeq 2.1 \times 10^{12}$ K.
Further more, for the parameters adopted in the present paper for 100 MHz and 13 kHz, for the fundamental emission, we obtain the brightness temperature, $T_B$ of $1.72 \times 10^8$ K and $7. \times 10^3 K$ if we assume the Rayleigh scattering as the emission mechanism, and $T_B$ of $3.06 \times 10^7$ K and $7.66 \times 10^8$ K if we assume the direct coupling as the emission mechanism, respectively, for the effective temperatures of the Langmuir critical fluctuations derived in this study.
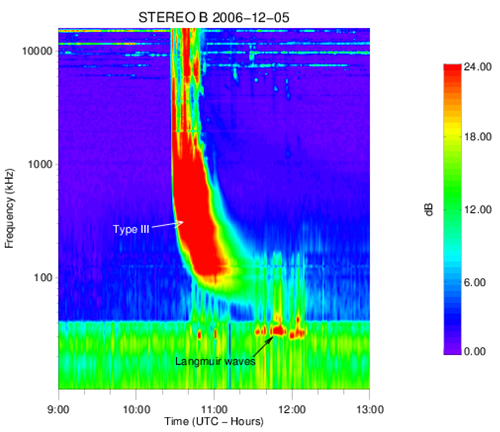
Figure 1: Dynamic Spectrum of a typical local solar type III radio burst (fast drifting emission from 5 MHz down to 32 kHz) and associated Langmuir waves (non-drifting emissions in the frequency interval 28-32 kHz) (Thejappa, MacDowall and Bergamo (2012))
As far as the electromagnetic emission at the second harmonic is concerned, if the generation mechanism is assumed to be the merging of beam excited Langmuir waves with back-scattered secondary Langmuir waves, we obtain $T_B \simeq 3. \times 10^{10}$ K and $3.1 \times 10^5$ K at 100 MHz and 13 kHz respectively.
Concluding Remarks
We conclude that: (1) the phenomenon of critical fluctuations in the beam-plasma system may be very important for type III bursts, (2) the ratio of beam to ambient electron density in weak type III bursts can be determined by the damping due to collisions in the corona, and by the damping due to scattering on density fluctuations in the Interplanetary medium, (3) since the instability does not develop in the case of critical fluctuations, the question of beam stabilization does not arise in the present model, (4) the direct coupling between Langmuir and electromagnetic waves appears to play a dominant role in conversion of Langmuir waves into electromagnetic waves at the fundamental plasma frequency, and (5) although the combination scattering is capable of explaining the emission at twice the plasma frequency, it is too inefficient to explain the type III bursts at lower frequencies.
Based on the recent paper: Thejappa, G., and MacDowall, R. J., Critical Fluctuations in Beam-plasma Systems and solar type III radio bursts, 2017, 16th AIAC: “Turbulence, Structures, and Particle Acceleration”, J. Phys.: Conf. Conf. Series 900 (2017) 012019, http://dx.doi.org/10.1088/1742-6596/900/1/012019
References:
Ginzburg, V. L., and Zheleznyakov, V. V., 1959, Soviet Astronomy, 3, 235.
Thejappa, G., MacDowall, R. J., and Bergamo, M, 2012, Journal of Geophysical Research: Space Physics, 117, A8, A08111
