Radio sources observed in the decametric range during type II and type III solar radio bursts are believed to be produced by coherent plasma emission due to electrostatic plasma oscillations induced by propagating suprathermal electrons (e.g. Ginzburg & Zhelezniakov 1958).
This type of emission is a valuable tool for observational diagnostics of the upper corona. Produced at the local plasma frequency, $f_{pe}\rm{[kHz]}= 8.93 (n_e\rm{[cm}^{-3}\rm{]})^{-1/2}$
or its harmonic, plasma emission can reveal the electron density structure of the upper corona. Early estimations of heliocentric distances of the sources in solar radio bursts showed they often appear further away from the Sun than expected from canonical coronal density models. This effect was interpreted as a result of enhanced density in the corona over the active regions (e.g. Wild et al. 1959). However, some argued that abnormal positions of the observed sources are due to scattering and refraction of radio-waves in the corona (e.g. Aubier et al. 1971). Indeed, the corona is not fully transparent for lower frequency radio waves: density inhomogeneities can scatter and refract propagating waves, affecting the apparent positions and sizes of the observed sources. Recently, McCauley et al. (2018) showed that coronal density enhancement cannot fully explain positions of radio sources in some type III radio-bursts observed with the MWA radio array in the range 80-240 MHz. Another recent work, by Chrysaphi et al. (2018), demonstrated that apparent positions of solar radio sources in a type II burst observed by LOFAR are consistent with the presence of strong radio-wave scattering. The aim of this study is to evaluate heliocentric distances of solar radio-sources at different frequencies, and compare them with the positions predicted by the Newkirk coronal density model.
Results
We use LOFAR observations of 12 different solar sources in the frequency range 30-48 MHz. Positional measurements are calibrated using several Tau A observations performed with the same observational set up. Calibration shows that, after applying the ionospheric refraction correction, the error of measurements of centroid position is 150 arcsec or less (Figure 1), which is applied as an error value for all solar observations at all frequencies.
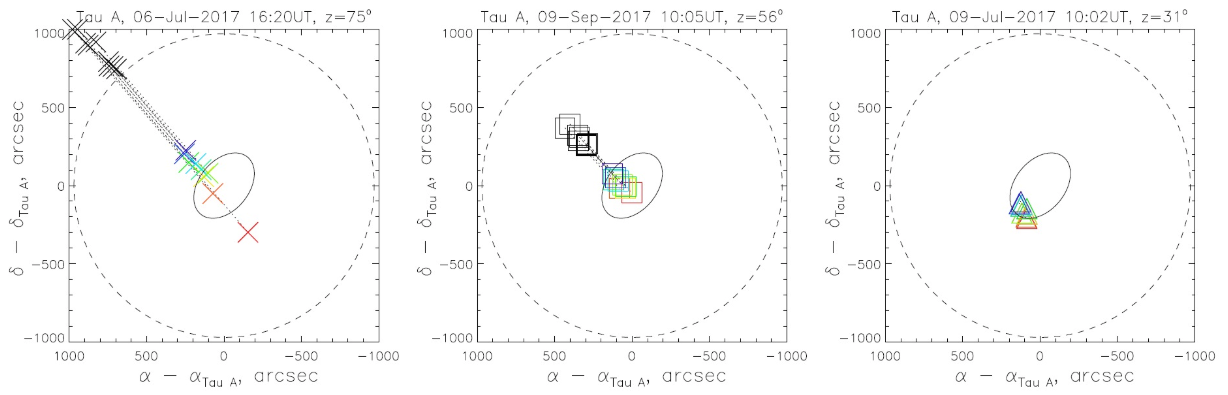Figure 1. Centroid positions of Tau A observed at different zenith angles at different frequencies. Black symbols show actual positions, colour symbols show positions corrected for ionospheric refraction. Different colours correspond to different frequencies, from 30 MHz (red) to 48 MHz (blue).
Our analysis shows that 3 of the 12 observed solar sources appear at heliocentric distances, which are substantially larger than those predicted by the Newkirk density model (Figure 2) or other canonical models (see Gordovskyy et al. 2019). The projection effect cannot explain these abnormal distances: non-90o projection angles would mean that the sources are located even further away from the Sun. There are three possible explanations for the observed effect: enhanced coronal densities, scattering and refraction of the radio-waves, and harmonic radio emission. The latter is very unlikely: in two of the “abnormal” sources emission is strongly polarised, with the polarisation degree up to 70-80%, which is inconsistent with the harmonic emission. Scattering of radio-waves in the turbulent corona can “shift” the apparent source positions by the observed value (0.2-0.7 Rsun), although it is not clear whether it can explain the observed flattening of the frequency-distance functions. The latter, however, can be explained by the density changes in the corona: active events in the corona should result in fast plasma motions and evaporation, which, in turn, would enhance the coronal density and reduce stratification. Enhanced hydrodynamic scale length in the upper corona would explain the flattening of the frequency-distance functions for the observed sources.
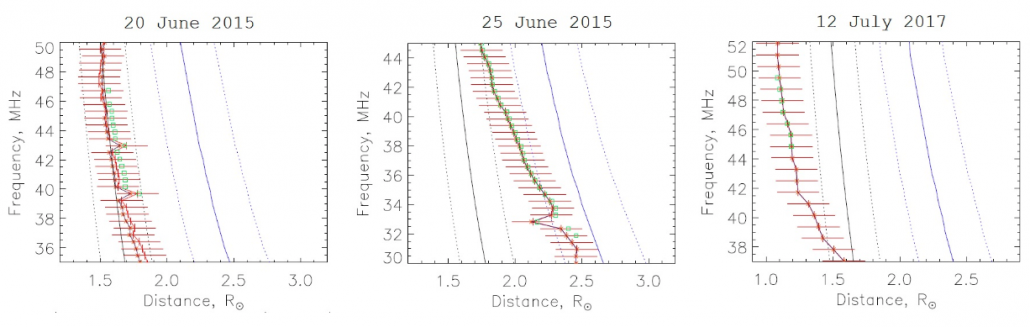Figure 2. Frequency-distance functions for three “abnormal” sources. Symbols show apparent source positions with the error bars. Solid black lines shows positions predicted by the Newkirk density model. Solid blue lines show positions predicted by the Newkirk model in presence of strong turbulence. Corresponding dotted lines show positions predicted by the densities x2 higher and lower than the mean densities (solid lines).
One of the “abnormal” events, observed on 25 June 2015, has been previously studied by Chrysaphi et al. (2018). They analysed this event at ~10:45UT, when its dynamic spectrum revealed band-splitting, indicating the presence of a shock. We, however, consider a different stage of this event, observed approximately 1 hour later, consisting of numerous type-III-like bursts (Figure 3). Interestingly, both studies yield very similar frequency-distance diagrams. This makes the radio-wave scattering in the corona the most viable interpretation for the observed effect: indeed, in the presence of strong scattering, apparent heliocentric distances to the sources should depend mostly on the characteristics of plasma turbulence, while the intrinsic positions of the sources are not important.
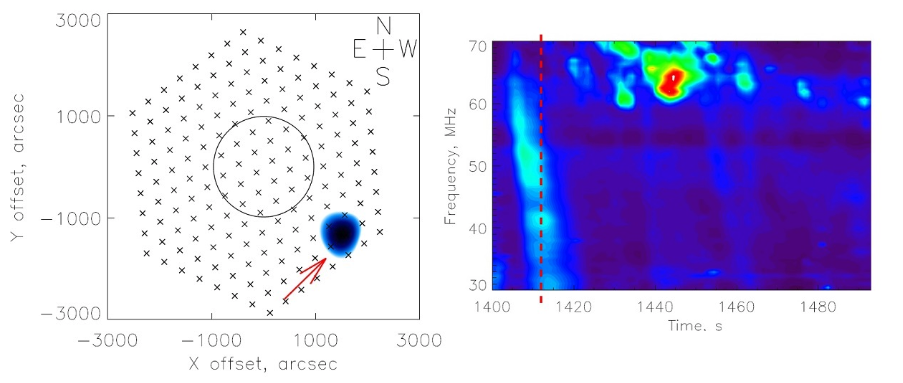
Figure 3. The dynamic spectrum (left) and the intensity map (right) for the 25 June 2015 event observed at 12:08UT.
Conclusions Based on our analysis, we conclude that the observed abnormal locations of solar radio-sources observed by LOFAR are likely to be caused by strong radio-wave scattering due to plasma turbulence in the active corona, as well as the enhanced coronal density.
Based on the recent paper by M.Gordovskyy, E.P. Kontar, P.K. Browning and A.A. Kuznetsov “Frequency-Distance Structure of Solar Radio Sources Observed by LOFAR”, 2019, Astrophysical Journal, 873, 48, doi: 10.3847/1538-4357/ab03d8
References
Aubier, M., Leblanc, Y. and Boischot, A., 1971, A&A, 12, 435.
Chrysaphi, N., Kontar, E.P., Holman, G.D. and Temmer, M., 2018, ApJ, 868, 79.
Ginzburg, V.L., Zhelezniakov, V.V., 1958, Sov.Astr, 2, 653.
McCauley, P.I., Cairns, I.H. and Morgan, J., 2018, Sol.Phys., 293, 132.
Wild, J.P., Sheridan, K.V. and Neylan, A.A., 1959, Aust.J.Phys., 12, 369.
Full list of authors: Mykola Gordovskyy, Eduard Kontar, Philippa Browning and Alexey Kuznetsov.